
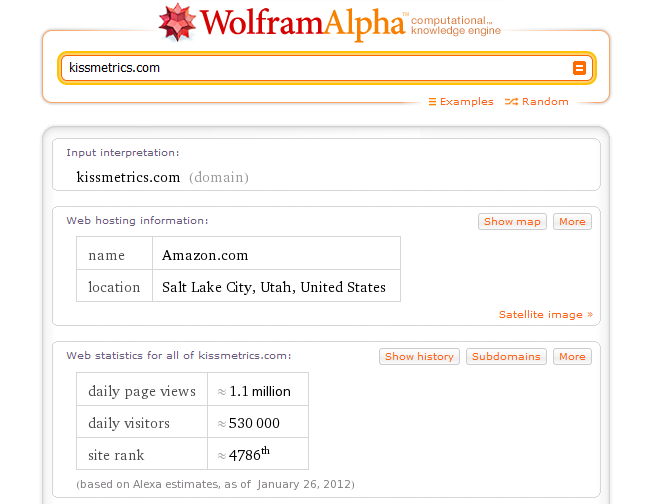
Such an assertion can then be used in functions such as Probability, NProbability, Expectation, and NExpectation. Distributed ], written more concisely as x LogNormalDistribution, can be used to assert that a random variable x is distributed according to a lognormal distribution. RandomVariate can be used to give one or more machine- or arbitrary-precision (the latter via the WorkingPrecision option) pseudorandom variates from a lognormal distribution.In addition, the lognormal distribution has become a widely utilized tool for modeling various phenomena, including dust concentrations, gold and uranium grades, flood flows, lifetime distributions for manufactured products, and miscellaneous phenomena in finance and economics. The theory of the distribution was studied further in the early 1900s and has since been found to accurately model both the weights of humans and the sizes of computer files on a file system. The origins of the lognormal distribution can be traced to an observation made by Francis Galton in the 1870s demonstrating that the distribution modeling the logarithm of a product of a number of independent positive random variates tends to a standard NormalDistribution as the number of variates gets infinitely large. In other words, if is a random variable and (where denotes "is distributed as"), then. LogNormalDistribution is the distribution followed by the logarithm of a normally distributed random variable.(This behavior can be made quantitatively precise by analyzing the SurvivalFunction of the distribution.) The lognormal distribution is sometimes called the Galton distribution, the antilognormal distribution, or the Cobb –Douglas distribution.
#Wolframalpha normal distribution pdf#
In addition, the PDF of the lognormal distribution has tails that are "fat", in the sense that its PDF decreases algebraically rather than decreasing exponentially for large values of. a global maximum) or monotone decreasing with a potential singularity approaching the lower boundary of its domain.
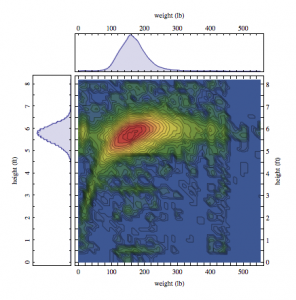
Depending on the values of σ and μ, the PDF of a lognormal distribution may be either unimodal with a single "peak" (i.e. LogNormalDistribution represents a continuous statistical distribution supported over the interval and parametrized by a real number μ and by a positive real number σ that together determine the overall shape of its probability density function (PDF).


 0 kommentar(er)
0 kommentar(er)
